by Lorenzo Audibert (EDF) and Houssem Haddar (Inria)
Wave imaging, a very useful technique for identifying remote/inaccessible objects using waves, is at the center of many widely used technologies such as RADAR, SONAR, medical imaging, nondestructive testing and seismic imaging. These techniques are well established for homogeneous backgrounds, such as air or water, for which a large variety of algorithms have been successfully used to solve the underlying imaging problem. However, most classical methods are inadequate at dealing with heterogeneous backgrounds, such as high resolution seismic imaging, ultrasound of bones, radar in urban environments and nondestructive testing of concrete or periodic nano-materials.
Wave imaging falls within the field of inverse problems, which is centred on how to stably invert an operator or a model that does not have a continuous inverse. This problem may be tackled in two ways: either by trying to obtain a complete description of the physical parameters of the probed medium or trying only to reconstruct its geometry. The first route would require a large amount of data and high computational cost, which may be prohibitive for real-time imaging, but very efficient methods can be designed by focussing on the geometrical support.
A recent framework for imaging the geometrical support of an object from measurements of scattered waves is offered by ‘sampling methods’ [1]. The idea of this class of methods is to build an indicator function that determines whether a given point is inside the scattering object or not, and this is done using only the range of the matrix formed by multi-sources multi-receivers sensing system, at a given frequency also called the measurement operator. For our method, developed as part of Lorenzo Audibert’s PhD thesis, a sampling point only lies inside if one can construct incident waves (by shaping the weight for each source) such that the medium scatters like a point source located at the sampling point. The main difficulty in the construction of incident waves is that the measurement operator, is extremely ill-conditioned. We propose the incorporation of a non-standard penalty term that controls a norm for the solution weighted by the measurement operator itself. A rigorous mathematical justification is given in [3] for the obtained algorithm that treats the case of noisy measurements and can handle various scenarios for collecting the data (near/far fields, full/limited apertures). The numerical accuracy of our new algorithms outperforms the accuracy of other existing sampling methods for noisy measurements [3].
The extension of those methods to complex media is an active topic in wave imaging. A commonly used approach for these problems is to exploit some asymptotic regimes (e.g., homogenisation, high frequency asymptotics, Born approximation) to get to a simpler setting. Sometimes, however, it is impossible to approximate any quantities that are related to the complex medium. In this seemingly impossible configuration, the use of two sets of measurements, one without and one with a perturbation, would provide enough information to be able to image modifications of the medium; this is known as ‘differential measurements imaging’. A typical problem is to locate changes in a medium without knowing precisely how this medium is structured and also in some cases the type of defect itself. Again we would like to design an imaging functional that only retrieves the geometrical information for efficiency purposes. Our algorithm is based on applying the sampling procedure to each set of data independently with the same carefully chosen simple artificial background. Indeed the obtained images are not expected to reveal the complex geometry of the true background and therefore the presence of modifications (due to other effects than noise in the data).
Inspired by the analysis of the sampling method above we propose to filter the difference between two solutions (defined as minimiser of the cost functional, introduced above) for a given sampling point. The filter is built from the data associated with defect free configuration. We prove that this difference eliminates the true background contribution and therefore reveals the presence or absence of defects and images its shape [2]. In terms of computations, we retain the distributed structure, and the computational cost for one sampling point is double that of the sampling method for an homogeneous background.
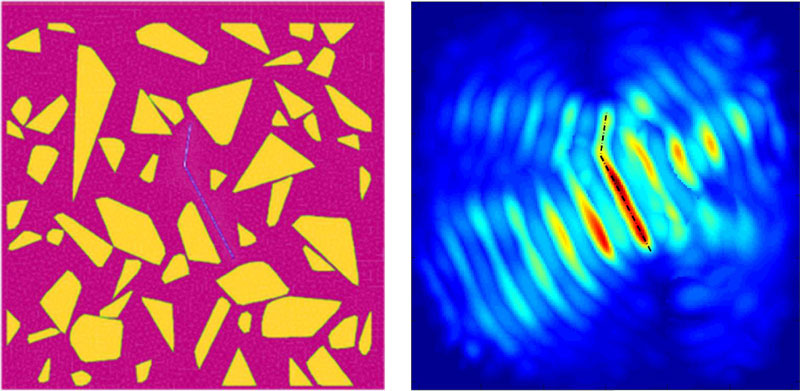
Figure 1: Differential imaging for retrieving a crack in concrete type material. Left: exact geometry – Right: obtained reconstruction using differential measurements.
We applied this method on synthetic data for ultrasound imaging in concrete type materials with the aim of detecting early stage cracks. Concrete can be seen as a two phase material; a large number of aggregates of various form and size bound together by cement paste. Cement paste is also a heterogeneous material but at the wavelength of ultrasound we can approximate it by a homogeneous media that is considered in our method as the reference background. Figure 1 shows the reconstruction for a crack inside a simulated concrete-like structure. Differential imaging is also being developed in order to image defects in periodic backgrounds (such as nanostructures) where for instance the periodicity information is exploited to avoid the need for a reference set of measurements.
Link:
[L1] https://pastel.archives-ouvertes.fr/tel-01203647
References:
[1] F. Cakoni, D. Colton, H. Haddar: “Inverse Scattering Theory and Transmission Eigenvalues”, SIAM publications, 88, 2016, CBMS Series.
[2] L. Audibert, G. Alexandre, H. Haddar: “Identifying defects in an unknown background using differential measurements”, Inverse Probl. Imaging, 2015.
[3] L. Audibert, H. Haddar: “A generalized formulation of the Linear Sampling Method with exact characterization of targets in terms of farfield measurements”, Inverse Problems, 2014.
Please contact:
Houssem Haddar, Inria, France











