Mathematical Models for the Conservation of Cultural Heritage

by Fabrizio Clarelli, Antonio Fasano, and Roberto Natalini

Air pollution is one of the most serious causes of degradation in carbonate stone, which in the form of marble, limestone and similar materials comprises some of the most important monuments and artefacts in the world. Mathematicians have now begun to model the degradation problem in order to assist in the protection and restoration of this stone. This paper presents a free boundary model, which describes the growth of a gypsum crust on the surface of marble monuments under SO2 aggression, and quantifies the influence of environmental factors such as pollutant concentration, humidity and temperature.

The list of great monuments and frescoes destroyed or seriously damaged by negligence or criminal action is dramatically increasing; sadly, some of the most lethal attacks have been from air pollution. Although pollution in European urban areas has decreased considerably in recent years, pollutants such as sulfur dioxide (SO₂) and nitrogen oxides (NO_x) are still present and active. SO₂ is the most important factor in the chemical deterioration of calcareous stones. It reacts with calcium carbonate to produce an external layer of gypsum (calcium sulfate) that eventually exfoliates (see Figures 1 and 2). The rate at which this process occurs depends greatly on the nature of the stone and the presence of moisture. Since the stone is porous, condensation of moisture may occur in pores deep inside the material. The reactivity of the material to the pollutant therefore becomes the critical factor.

Figure 1: Head of a rain god, carved on the Antonina column in Rome. Erosion and crusts have been caused by SO2 pollution.

Despite intense experimental research by conservation scientists, further studies are necessary in order to provide a predictive tool. Chemistry, of course, has the most to reveal about the causes of stone degradation. However, mathematical modelling can lead us to a better understanding of the relative importance of the simultaneous processes involved. Models can help to predict the evolution of phenomena and their dependence on seasonal variations in basic physical quantities (eg air pressure, temperature, moisture content, rainfall and - naturally - pollutant concentration). As a consequence, a mathematical model can be used as the basis for determining an optimal strategy for restoration or even prevention.

These problems are currently being considered by groups working at the Istituto per le Applicazioni del Calcolo 'M. Picone' (IAC-CNR) in Rome, in collaboration with a group from the Department of Mathematics at the University of Florence. Using an initially simplified approach, differential models of the evolution of the gypsum crust have been proposed, and then validated against laboratory experiments.

Figure 2: SEM-EDS map of the chemical elements on a marble surface exposed for 144 hours to SO2 aggression (calcium carbonate: on the left; gypsum: on the right). Data obtained in a laboratory test (courtesy of M.L. Santarelli of CiSTEC, Rome).

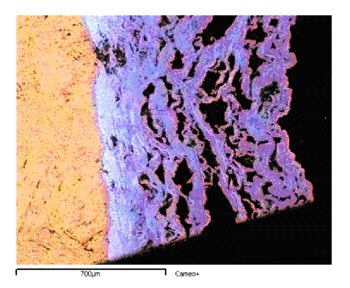
More recently, we have developed a more realistic free-boundary model, and investigated it in detail (see link below). This model includes important features such as swelling and relative humidity. These two factors have a strong influence on the evolution of sulfation and therefore demand specific consideration. The transformation of marble into gypsum is accompanied by a volume change, which yields quite a consistent swelling. Although determining the swelling rate is not easy, it is reasonable to say that the volume of the gypsum produced during the transformation is two or three times the volume of pristine marble. Concerning humidity, and taking a lead from the chemical literature, we have determined the existence of two different regimes for the growth rate of the gypsum crust according to the relative humidity.

Figure 3: Simulation of the evolution in time (over one1 year) of gypsum formation, with clearing after six months (blue line) and without clearing (red line). We used real data taken at Villa Ada in Rome (courtesy of Arpalazio, Agenzia Regionale per la Protezione Ambientale).

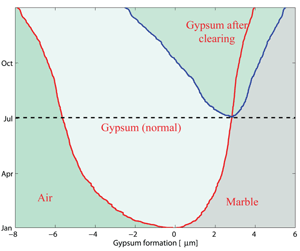
Using this new model, simulations have been performed with real data acquired at Villa Ada in Rome and kindly provided by Arpalazio, the Rome regional authority for monitoring pollution. Useful indications were derived by our elaboration of these data. First, the growth of the crust is proportional to √t (where t is time). For instance, using Villa Ada data we have extrapolated from the experimental data of the first year (2006) to the following eight years. After one year, we obtain a crust of 4µm, with 8.2µm after four years and 12.5µm after nine years. This result allows us to quantify the consequence of crust removal after a given time (see Figure 3). Next, we established the influence of the variation of SO₂ by a factor c. The external concentration of SO₂, s(t), was replaced by the value c.s(t), where c is a given constant. We again used ‘Villa Ada’ data, and simulated the front behaviour according to the different values of c. The results obtained show that the thickness of the front varies as √c . So, even if we were able to diminish the concentration of SO₂ pollutant by four times, we would only obtain a reduction of one-half of marble waste.

We are now starting a collaboration with the General Direction of Cultural Heritage in Latium and with the ‘Istituto Centrale per il Restauro’ in order to undertake more significant tests of our approach.

Links:
http://www.iac.rm.cnr.it/~natalini
http://www.iac.rm.cnr.it/~natalini/ps/CFNsulf.pdf
http://web.math.unifi.it/users/fasano/home.html

Please contact:
Roberto Natalini
IAC-CNR, Italy
Tel: +39 06 88470257
E-mail: r.nataliniiac.cnr.it